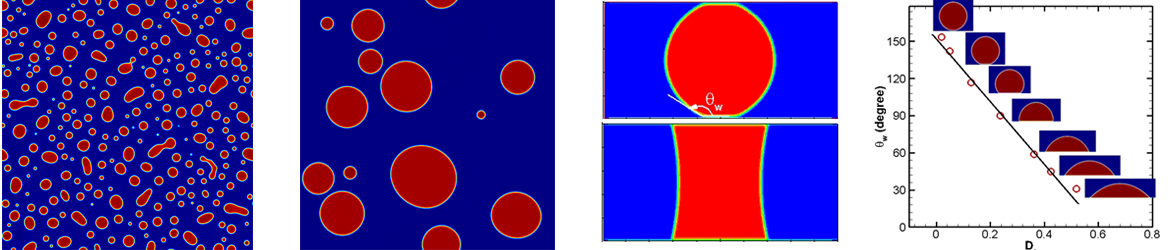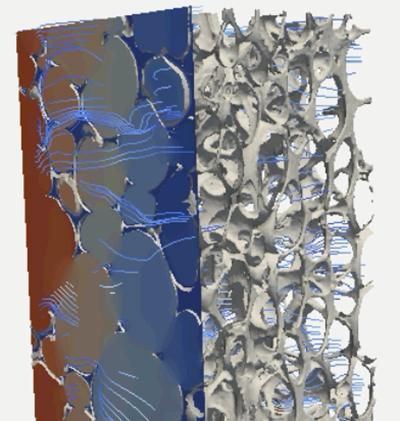
Porous Media
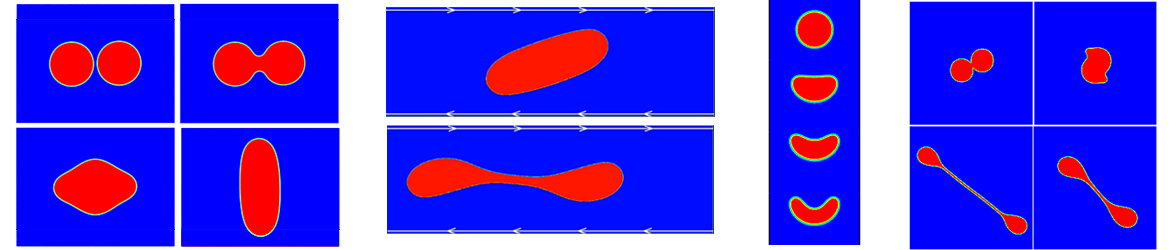
Natural and manmade materials such as soils, rocks, concrete, foams and even biological tissues can be studied as porous media. Flow and transport in porous media have many applications in nature science and industry. The area of interest of poromechanics was initially limited to more classical topics of soil and reservoir mechanics such as consolidation, land subsidence, slope stability, fault slippage, reservoir compaction and dam engineering but then there was an explosive expansion involving pumping and injection induced seismicity, outburst of coal formation, hydraulic fracturing, water wave and sediment interaction, drying of inkjet-printed droplets on paper, aquifer response to tidal and barometric loading, thermal fracturing and burst of concrete cylinder, swelling of clay and shale, and seismoelectric and seismoelectromagnetic phenomena, biomedical applications such as squeeze film lubrication of articular cartilage, healthy and diabetic foot behavior, tumor growth, glaucoma modeling and others. Our research efforts focus on the fundamental understanding of multiphase flow pattern and relevant phenomena as well as flow transport through the porous media. We address the thermo-hydro-mechanical aspects of porous environment and intend to decipher the links between particle-level scale and macroscale behavior. Our findings will be significant to applications in aerospace, mechanical, mining and petroleum engineering, environmental engineering and biomedical technology.
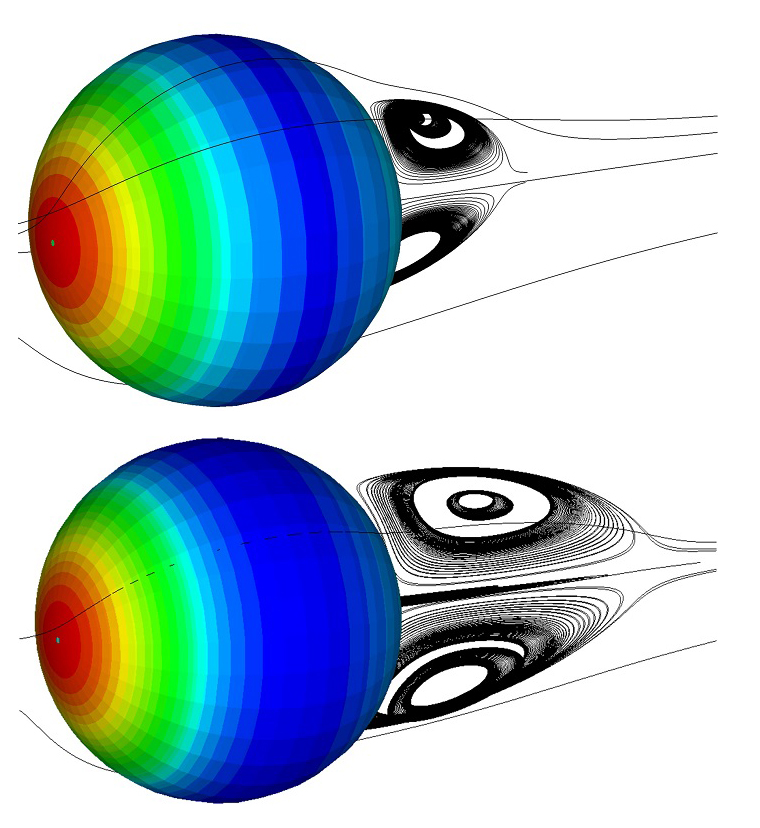
Cavitation
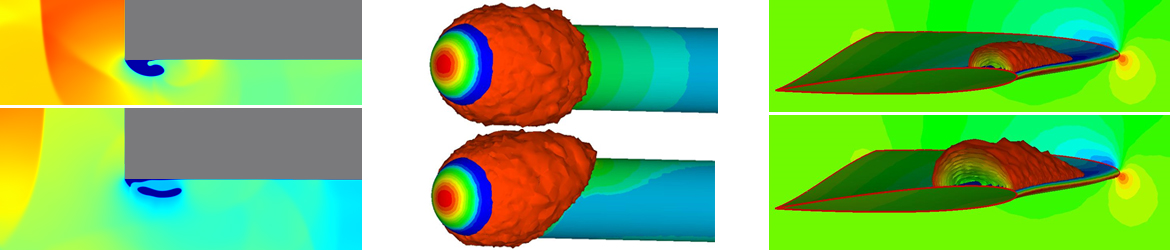
Cavitation is defined as the formation of the vapor phase in a liquid due to the reduction of pressure to some critical value at approximately constant temperature. The term cavitation can imply anything from the initial formation of bubbles (inception) to large-scale, attached cavities (supercavitation). Any device handling liquids is subject to cavitation. Negative effects of cavitation include performance degradation in turbomachinery and propulsion systems, noise and vibration and cavitation erosion. Useful applications of cavitation applications include ultrasonic cleaning, the homogenization of milk. Various chemical processes are enhanced by cavitation, such as coagulation, formation of suspensions and degassing of liquids. Cavitation can be used to increase heat and mass transfer in liquids, to promote crystallization and to enhance various sonochemical reactions such as polymerization and polymer degradation. Biomedical applications include the removal of kidney stones and automated drug delivery to patients. Important new applications in the pollution control area are also of interest.
The Mutiphase Flow Laboratory (MPFL) has a deep research activity in this field as well as extensive engineering experience in various applications. Most of our research is conducted in an interactive experimental/numerical/analytical approach. Fundamental research includes cavitation inception studies, super-cavitating and ventilated hydrofoil theory, drag reduction through super- or partial cavitation and microbubbles, effect of cavitation on fluid transients, vortex cavitation, sheet/cloud cavitation, cavitation induced lift oscillations, effects on fluid transients cavitation erosion, and acoustics of bubbly flows, and many other related topics. The laboratory pioneered the development of cavitation nuclei measurement based on the multiphase lattice Boltzmann method. At the same time, related efforts in the development of 3D, unsteady flow computational models was applied to sophisticated computer codes for calculating cavitating flow in complex geometry. As progress was made in computational fluid dynamics, the emphasis of this research has shifted from exclusively experimental and analytical studies to the use of an interactive experimental/numerical approach.
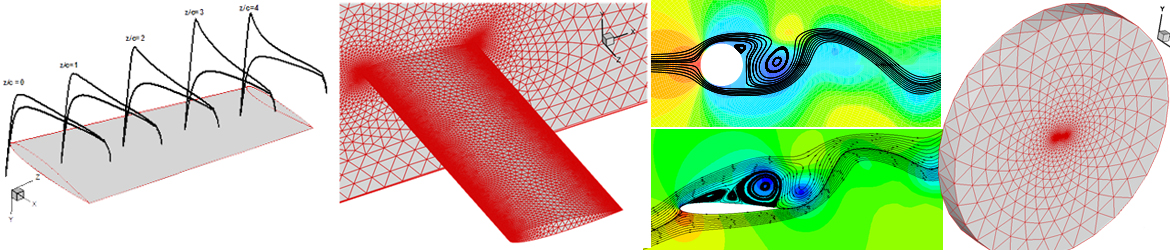
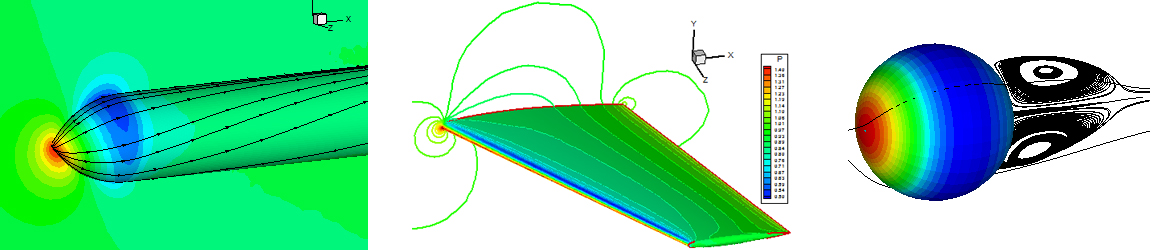
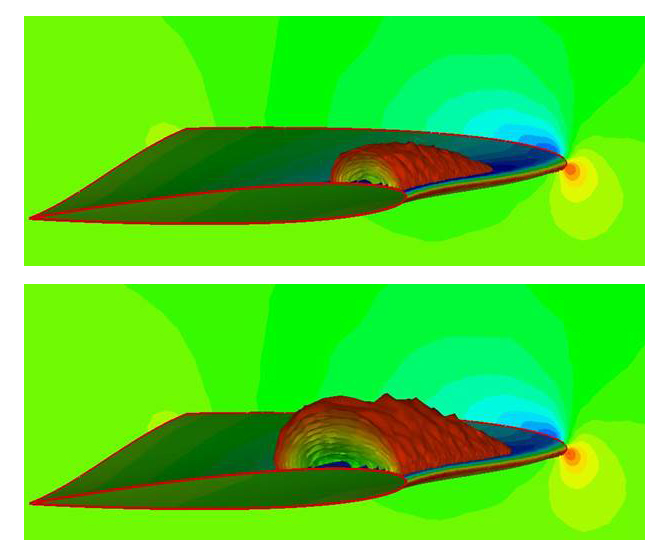
Computational Aero&Hydrodynamics
Our group has most up-to-date and comprehensive experiences to provide all levels of aerodynamic and hydrodynamic design and analysis ranging from basic engineering methods to advanced computational approaches. At the core of the research, novel computational solvers are developed by the group. An efficient and parallel Reynolds-averaged Navier Stokes flow solver as well as an accurate algorithm based on the high-order lattice Boltzmann method provide two unique and powerful approaches for simulation of several applied Aero&Hydrodynamic problems at wide range of flow conditions. Our current research in this area concentrates on algorithm development, aerodynamic and hydrodynamic flow control, shape optimization and drag reduction.